The Epistemics of First Principles

Paul DeHart recently published at Public Discourse an excellent essay arguing that natural-law moral systems in the Aristotelian-Thomistic tradition do not involve the so-called naturalistic fallacy, the supposed mistake of inferring an ought from an is. He was responding to a piece by F.H. Buckley, and I agree with all DeHart’s major points.

One matter in DeHart’s essay, however, bears further explanation, for he seems to give two incompatible accounts of moral first principles. For, at one point, DeHart says that the first principles of morality can be justified on the basis of human nature and the human function: “an ought can be inferred from an is in a metaphysic in which the function of something is part of its nature.” This is the standard move in Aristotelian-Thomistic moral systems going back to the Nicomachean Ethics, and I agree it is correct. Later, however, DeHart says that the first principles of morality are true but unprovable and must be accepted as given if there is to be any inquiry at all. The first principles “are the beginning of moral reasoning and, like geometric axioms, the basis of moral demonstration.” “We begin with them without arriving at them by a chain of reasoning.”

The contradiction here is only apparent. As Aquinas says, some disciplines accept their first principles from other, higher disciplines. When we are doing natural law, we are arguing about which laws or policies we morally ought to have. In such inquiries, we take the first principles of morals as given and argue about what they require in particular situations. Hence, DeHart says that these principles are “underived” and merely “stipulated in the major premise” of the natural-lawyer’s argument. But, if someone challenges one of these first principles and asks, say, how we know that murder is wrong, then the nature of the inquiry shifts. We are no longer doing morality but metaethics, the discipline that investigates the foundations of moral claims and how we know such claims are true. In this higher discipline, the first principles of natural law can be justified by arguments based on the nature shared by all members of the species homo sapiens, which implies an objective human function that allows us to evaluate human actions as being good or bad—that is, rationally ordered to the fulfillment of the human function or not. Thus, one discipline takes the principles of morality as given, and another justifies those principles.

DeHart knows all this, of course, but I worry that some people will misunderstand his analogy between morals and geometry. In particular, some natural-law theorists think that, in natural law, there are fundamental axioms, ultimate necessary truths, that we human beings immediately grasp as necessary truths when we hear them. Such principles, they say, are self-evident or per se nota the way sentences like “Every bachelor is unmarried” or “No part is greater than its whole” are said to be. On this view, all other true propositions of natural law follow from these necessary, self-evident axioms by pure logic.

This understanding of natural law is clearly false. If it were true, natural lawyers would produce formal proofs the way mathematicians do, and everyone would agree about which propositions of natural law had been proved, just as mathematicians agree about which theorems in geometry have been proved. But natural lawyers never produce formal proofs—indeed, they don’t even try to do so—and moral discourse is notorious for its seemingly intractable disagreements. However we come to know truths of natural law, the process is nothing like that in geometry.

Here I focus on one particular error in this natural-law-as-geometry account—the idea that there are necessary truths that we can recognize as such when we hear them. That is, I am not denying that there are necessary truths; there certainly are. I am denying that we human beings have an ability to recognize the necessary truths on sight, as it were. In particular, I want to explain that this idea is false even about geometry. The argument is then a fortiori: if we have no ability to recognize on sight necessary truths of geometry, where there is nearly universal agreement on such truths, then it is beyond implausible that we have such an ability with respect to morality or natural law, where there is profound disagreement. This requires that we talk about geometry, but bear with me, because the story involves some of most famous episodes in the history of science.

The Parallel Postulate in the History of Physics

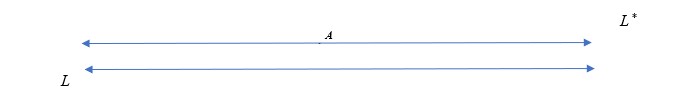
Everyone knows that, in Euclid’s geometry, theorems are deduced logically from a set of axioms. One of these axioms is the so-called Parallel Postulate, which says that for any point A not on a given line L,

there is one and only one line L ^* that passes through point A and does not intersect line L (this is actually Playfair’s reformulation of the Parallel Postulate, but it’s easier to understand than Euclid’s version):

For millennia mathematicians tried to prove the Parallel Postulate from Euclid’s other axioms, hoping that the postulate could be downgraded to a theorem. All of these efforts failed. Then, in the nineteenth century, something that had been literally unthinkable happened. Mathematicians began to consider systems in which the Parallel Postulate was false.

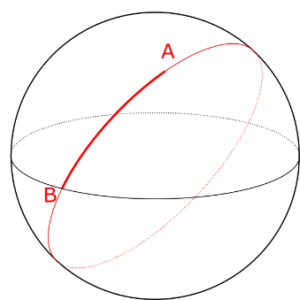
Does this lead to a contradiction? No, for if it did, the Parallel Postulate could have been proved from the other axioms after all by a reductio ad absurdum. When you assume the postulate is false, you get not a contradiction but different, perfectly sensible systems of geometry. For example, if you assume that, for any point not on a given line, every line through that point intersects the given line, you get elliptic geometry, which is the natural geometry of the surface of a sphere:

Another possibility is that, for a point not on a line, there are multiple lines through the point not intersecting the line; that assumption produces hyperbolic geometry. Euclid’s geometry thus no longer appears to be the one true geometry based on necessary truths but merely one system of geometry among many. Is Euclidean geometry special or privileged? No, except in the sense that it’s the system of geometry most readily understood by the human mind. In particular, Euclidean geometry is not truer than other geometries; each set of axioms is true in some models but not in others. Euclid’s axioms are true in so-called Euclidean spaces but false on the surface of spheres; for elliptic geometry, it’s the reverse. Moreover, there is no more relativism here than there is in saying “It’s raining” is true in London but false in San Diego.

Most surprising of all, one of the places Euclid’s axioms don’t hold is the actual physical universe. For Newton, the geometry of the physical universe was Euclidean, but in Einstein’s General Relativity, space is curved. That is, the geometry included in General Relativity is a hyperbolic, non-Euclidean one. So Euclidean geometry, so far from being necessarily true about the physical universe, is actually false.

Nor is the Parallel Postulate the only “necessary truth” that turns out to be false. Indeed, quantum mechanics calls into question an apparent necessary truth much more basic than the Parallel Postulate—a very law of logic. That is, in classical logic, conjunction distributes over disjunction: “P and (Q or R)” is logically equivalent to “(P and Q) or (P and R).” But all the mysterious anomalies in quantum mechanics—the impossibility of a particle having both a position and momentum at the same time, for instance—vanish if we give up this law of logic. For this reason, Birkhoff and von Neumann famously suggested that the true logic of the universe is not classical logic but a slightly different logic, now known as Quantum Logic, in which conjunction does not distribute over disjunction. This solution to the quantum anomalies is so radical that it has found few takers, but it remains a viable theory.

Indeed, even the Law of Non-Contradiction is not immune to possible revision. In so-called paraconsistent logics, a limited range of propositions can be both true and false. Why would anyone accept such a system? Well, consider the Liar Paradox, sentences like, “This sentence is false,” which is true if it’s false and false if it’s true. Classical logic, which includes the Law of Non-Contradiction, deals with such sentences by rejecting them as non-sentences; they are effectively declared ungrammatical. In some paraconsistent logics, such sentences are perfectly good sentences that are both true and false, adjustments being made to other logical axioms to cabin the contradiction to the liar-sentences and prevent the whole system from collapsing. I am not convinced that this is the best way to deal with liar sentences, but, like Quantum Logic, it’s not a crazy suggestion.

Our Knowledge of Necessary Truths

Does it follow that there are no necessary truths? Not at all. There are necessary truths, it is just that a sentence’s seeming to us to be necessary, its being “self-evident,” is no guarantee that the sentence is true, much less necessarily true. Like the Parallel Postulate, a sentence may seem for all the world to be necessary and then turn out to be false.

But if we cannot recognize necessary truths just by contemplating their terms, how do we come to know them? The answer is that we discover them empirically. For instance, Newton told us that certain sentences—his laws of physics, including the Parallel Postulate and the other axioms of Euclidean geometry—were necessary truths about the physical universe. In Newton’s day and for a long time thereafter, there were excellent reasons for thinking these sentences were indeed necessary truths, for Newtonian physics did a remarkable job of accurately predicting physical phenomena. But there were problems too, most notably that Newtonian physics predicts that the perihelion of Mercury should precess 5,600 seconds of arc per century, while the observed rate is only 5,557 seconds of arc per century—a small but measurable difference. Physicists invented explanations for the discrepancy (dust surrounding the sun was a popular one), but none of these worked out. Then came Einstein and his theory of General Relativity, which gets right everything that Newton got right and predicts the precession of the perihelion of Mercury exactly correctly—precisely 5,557 seconds of arc per century.

Based on such empirical evidence, we now think that Einstein’s theory is correct and that the sentences his theory identifies as necessary truths about the physical universe are indeed necessary truths. Those necessary truths include the axioms of non-Euclidean geometry that form an essential part of the theory. (In fact, the difference of 43 seconds of arc in the precession of the perihelion of Mercury arises from the very curvature of space in General Relativity’s non-Euclidean geometry.) If Quantum Logic turns out to be the best way to understand certain subatomic phenomena, then we will have good empirical evidence that the actual logic of the universe is not classical logic but Quantum Logic. In that case, we should say that, just as, in our human-sized world, Euclidean geometry seems true but really isn’t (its falsity becoming apparent only on astronomical scales), so too, in our human-sized world, classical logic seems true but really isn’t (its falsity becoming apparent only on the subatomic level). In both cases—axioms of geometry in the physical universe, laws of logic—there are indeed necessary truths, but we discover them empirically.

First Principles in Morality

The first principles of morality are no different. We discover empirically that human beings have certain objective properties. On this basis, we formulate an account of the human function, the final end or telos for which our objective properties suit us. The form of reasoning is just like that of an archeologist working out the function of an ancient artifact like the Antikythera Mechanism. From experience, we learn the natural effects of various kinds of human actions, and so we formulate principles about which actions are ordered as means to the final end (good actions) and which are not so ordered (bad actions). When we notice that a certain kind of action—say intentionally killing the innocent—is not ordered to the final end regardless of the circumstances in which it is performed, we have discovered a necessary truth of morality. But just as in physics, such truths are discovered empirically, not immediately grasped because they are self-evident.

Some readers will be disturbed by this conclusion, for if our knowledge of necessary first principles, whether in science or morality, is ultimately based on experience, we can never be quite certain that the statements we currently take for necessary truths really are necessary or even true. This is correct, but it shouldn’t be disturbing. Fallibility is part of the human condition. In this life, our knowledge is imperfect, whether of contingent things or necessary ones, whether in science or in morals.